Difference between revisions of "Shulbasutras (शुल्बसूत्राणि)"
(adding content under various headings and their references) |
(→Relationship between Shulba and Yajnas: adding content and references) |
||
| Line 12: | Line 12: | ||
=== List of Shulbasutra Works === | === List of Shulbasutra Works === | ||
| − | Of the available extant texts, Baudhayana Shulbasutra is considered to be the most ancient one. | + | Of the available extant texts, Baudhayana Shulbasutra is considered to be the most ancient one. Following is the list of shulbasutras and their commentators. |
{| class="wikitable" | {| class="wikitable" | ||
!Vedas | !Vedas | ||
| Line 118: | Line 118: | ||
The chitis had rich symbolic significance and their designs were often intricate. For instance, the Syenacit has the shape of a falcon in flight (a symbolic representation of the aspiration of soaring upward); the Kurmachit is shaped as a tortoise, with extended head and legs, the rathacakracit as a chariot wheel with spokes, and so on.<ref>A. K. Dutta and M. S. Sriram. ''[https://pdfs.semanticscholar.org/bb84/9b5ff23bc22e2056f0aab069c92c05f7af0c.pdf Mathematics and Astronomy in India before 300 BCE.]'' </ref> | The chitis had rich symbolic significance and their designs were often intricate. For instance, the Syenacit has the shape of a falcon in flight (a symbolic representation of the aspiration of soaring upward); the Kurmachit is shaped as a tortoise, with extended head and legs, the rathacakracit as a chariot wheel with spokes, and so on.<ref>A. K. Dutta and M. S. Sriram. ''[https://pdfs.semanticscholar.org/bb84/9b5ff23bc22e2056f0aab069c92c05f7af0c.pdf Mathematics and Astronomy in India before 300 BCE.]'' </ref> | ||
| + | |||
| + | == Subject-matter of Shulbasutras == | ||
| + | Baudhayana shulbasutras are considered to be the most ancient of the Shulbasutra texts. It also presents a very systematic and detailed treatment of several topics that are skipped in later texts. | ||
| + | {| class="wikitable" | ||
| + | |+Topics covered in Baudhayana Shulbasutra<ref name=":1" /> | ||
| + | ! | ||
| + | !Topics | ||
| + | !English Equivalent | ||
| + | |- | ||
| + | |1 | ||
| + | |रेखामानपरिभाषा | ||
| + | |Units of linear measurement | ||
| + | |- | ||
| + | |2 | ||
| + | |चतुरश्रकरणोपायः | ||
| + | |Construction of squares, rectangles, etc | ||
| + | |- | ||
| + | |3 | ||
| + | |करण्यानयनम् | ||
| + | |Obtaining the surds/theorem of the square og the diagonal | ||
| + | |- | ||
| + | |4 | ||
| + | |क्षेत्राकारपरिणामः | ||
| + | |Transformation of geometrical figures | ||
| + | |- | ||
| + | |5 | ||
| + | |नानाविधवेदिविहरणम् | ||
| + | |Plan for different sacrificial grounds (darsa, pasubandha, sutramani, agnistoma etc) | ||
| + | |- | ||
| + | |6 | ||
| + | |अग्नीनां प्रमाणक्षेत्रमानम् | ||
| + | |Areas of the sacrificial agnis/vedis | ||
| + | |- | ||
| + | |7 | ||
| + | |इष्टकसङ्ख्यापरिमाणादिकथनम् | ||
| + | |Specifying the number of bricks used on the construction of vedis including their sizes and shapes | ||
| + | |- | ||
| + | |8 | ||
| + | |इष्टकोपधाने रीत्यादिनिर्णयः | ||
| + | |Choosing clay, sand, etc in making bricks | ||
| + | |- | ||
| + | |9 | ||
| + | |इष्टकोपधानप्रकारः | ||
| + | |Process of manufacturing the bricks | ||
| + | |- | ||
| + | |10 | ||
| + | |श्येनचिदाद्याकारनिरुपणम् | ||
| + | |Describing the shapes of syenachiti | ||
| + | |} | ||
| + | A brief explanation of a few topics dealt in the shulbasutras is given below | ||
| + | |||
| + | === Approximation of Surds === | ||
| + | Besides presenting the details related to the construction of altars - that generally possess a bilateral symmetry - the Shulbasutra texts also present different interesting approximations for surds. The motivation for presenting estimates of surds could be traced to the attempts of vedic priests | ||
| + | * to solve the problem of 'squaring a circle' and vice versa | ||
| + | * to construct a square whose area is n times the area of a given square, and so on | ||
| + | |||
| + | === Determination of cardinal directions === | ||
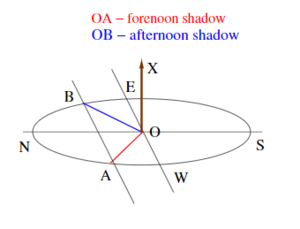
| + | [[File:Determining Cardinal Directions.PNG|thumb|Determination of Cardinal Directions]] | ||
| + | Determining the exact east-west line at a given location is a prerequisite for all constructions, be it a residence, a temple, a vedi or yajna kunda. Finding the cardinal directions using a shanku has been described thus:<ref name=":1" /><blockquote>समे शंङ्कु निखाय शंङ्कुसम्मितया रज्ज्वा मण्डलं परिलिख्य यत्र लेखयोः शंङ्क्वग्रच्छाया निपतति तत्र शंङ्कू निहन्ति सा प्राची । (Katy. Shul. Sutr. 1.2)</blockquote>Fixing a pin (or gnomon) on levelled ground and drawing a circle with a cord measured by the gnomon, he fixes pins at points on the line of the circumference where the shadow of the tip of the gnomon falls. That gives the east-west line (prachi). | ||
| + | |||
| + | Now, to answer the question about the necessity of such an experiment, instead of simply looking at the sunrise or sunset to determine the cardinal directions, the commentator Mahidhara observes:<blockquote>... तस्य उदयस्थानानां बहुत्वात् प्रतिदिनं भिन्नत्वात् अनियमेन प्राची ज्ञातुं न शक्या। तस्मात् शङ्कुस्थापनेन प्राचीसाधनमुक्तम्। दक्षिणायने चित्रापर्यन्तमर्कोऽभ्युदेति। मेषतुलासङ्क्रात्यहे प्राच्यां शुद्धायामुदेति। ततोऽर्कात् प्रचीज्ञानं दुर्घटम्।</blockquote>Meaning: Since the rising points are many, varying from day to day, the (cardinal) east point cannot be known (from the sunrise point). Therefore it has been prescribed that the east be determined by fixing a Shanku (शङ्कु)... therefore simply looking at the sun and determining the east is difficult. | ||
| + | |||
| + | Having obtained the prachi, getting udichi (the north-south line) correctly is extremely important for the construction of various vedis having bilateral symmetry.<ref name=":1" /> | ||
== References == | == References == | ||
Revision as of 18:55, 27 March 2020
| This article needs editing.
Add and improvise the content from reliable sources. |
Shulbasutras (Samskrit: शुल्बसूत्राणि) are manuals for the construction of yajnas. They are sections of the Kalpasutras, associated in particular with the Shrautasutras. Each Shrautasutra seemed to have their own Shulbasutra section based on literary data; however, in the present days, only seven of these sutra works, Baudhayana, Apastamba, Katyayana, Manava, Maitayana, Varaha and Vadhula are available.
Introduction
Recognized as the oldest and earliest treatises on mathematical problems, Shulbasutras give us a glimpse of the knowledge of geometry that the vedic people possessed. Incidentally they furnish us with a few other subjects of much mathematical interest.[1]
One of the prime occupations of the vedic people, performing yajnas, required altars or yajna-vedis of prescribed shapes and sizes. It was primarily in connection with the construction of altars that problems of geometry and also of arithmetic and algebra presented themselves to these ancient rshis, which led to the development of these texts.
Authors and Commentators
Unlike the other Sutra charanas, there are a very few Shulbasutra texts available at present and all of them belong to Yajurveda. The Shulbasutras of Baudhayana, Apastamba, Katyayana and Manava are the main sources of the knowledge of geometry. The Kalpa texts of Vedangas clearly outline that astronomy on one hand and geometry on the other were cultivated under different circumstances by different rtviks based on the duties apportioned to them in the conduct of yajnas.[2][1]
List of Shulbasutra Works
Of the available extant texts, Baudhayana Shulbasutra is considered to be the most ancient one. Following is the list of shulbasutras and their commentators.
| Vedas | Shakas | Shulbasutra | Contents | Commentators |
|---|---|---|---|---|
| Rigveda | Shakala | None available | ||
| Kaushitaki | ||||
| Shukla Yajurveda | Vajasaneya | Katyayana | Purvabhaga (7 Kandikas with 90 Sutras)
Uttarabhaga (40 or 48 shlokas) |
Rama or Ramachandra (Shulba-sutra-vrtti), Shulbasutravivarana (Mahidhara), Karka (Shulbasutrabhashya) |
| Krishna Yajurveda | Taittriya | Baudhayana | 3 Adhyayas (about 520 Sutras) | Dvarakanatha Yajva (Sulba-dipika), Venkatesvara Dikshita (Shulba-mimamsa) |
| Apastamba | 6 Patalas (21 Adhyayas and 223 Sutras) | Kapardisvami (Shulba-vyakhya), Karavindasvami (Shulba-pradipika), Sundararaja (Shulba-pradipa or Sundararajiya), Gopala (Shulbabhashya) | ||
| Varaha | 3 Adhyayas | |||
| Hiranyakeshi | ||||
| Vadhula | Vadhula (वाधूलः) | |||
| Maitrayani | Manava by Manu | 7 Khandas | Narada | |
| Maitrayana | 4 Khandas | Shankarabhatta | ||
| Samaveda | Kauthuma | None available | ||
| Rananiya | ||||
| Jaimini | ||||
| Atharvaveda | None available | |||
Qualities of a Shulbakara
Mahidhara in his commentary on Katyayana shulbasutra succintly describes the qualities of a Shulbakara.[3]
सङ्ख्याज्ञः परिमाणज्ञः समसूत्रनिरञ्छकः । समसूत्रौ भवेद्विद्वान् शुल्बवित् परिपृच्छकः।।
शास्त्रबुद्धिविभागज्ञः परशास्त्रकुतूहलः। शिल्पिभ्यः स्थपतिभ्यश्चाप्याददीत मतीः सदा।।
तिर्यङ्मान्याश्च सर्वार्थः पार्श्वमान्याश्च योगवित्। करणीनां विभागज्ञः नित्योद्युक्तश्च सर्वदा।।
A shulbakara must be versed in arithmetic, versed in mensuration, must be an inquirer, quite knowledgeable in one's own discipline, must be enthusiastic in learning other disciplines, always willing to learn from practicing sculptors and architects... and one who is always industrious.
Etymology
The word Shulba (शुल्ब) means a 'cord', 'a rope', 'a string'. A Sutra refers to a short rule. The commentators refer to this subject matter as Shulba as the true name, Shulba-parishista, Shulbi Kriya etc.
In few other instances the word "rajju (रज्जुः) has been used. B. B. Datta states that the words "shulba (शुल्ब)" "shulva (शुल्व)" and "rajju (रज्जुः) may be used synonymously to mean a rope or cord.[1]
The word "shulba or shulva" (sulva) stems from the dhatu "शुल्ब्" used in the meaning "माने । to measure" and hence its etymological significance is "measuring" or act of measurement. The term Shulba can be presented in the following ways[3]
- भावव्युत्पत्तिः - शुल्बनम् इति शुल्बः । the act of measuring (mensuration)
- कर्मव्युत्पत्तिः - शुल्बयते इति शुल्बः । the entity/result obtained by measuring (line)
- करणव्युत्पत्तिः - शुल्बयत्यनेन इति शुल्बः । the instrument of measuring
In the Shulbas the measuring tape is called "Rajju", so is "a line". One who is well-versed in the science of Shulba (geometry) is called Shulbavijnana, a Shulbavid (expert in geometry), a Sulbapariprcchaka (an inquirer into the Shulba), a Samkhyaajna (expert in numbers), Parimaanajna (expert in measuring). A special term sama-sutra-niranchaka (meaning uniform rope stretcher) is also used, this rope plays a very significant role and we find many references of similar words rajjuka, rakku-grahaka, sutra-grahaka - all used to denote a maharaja's land surveyor. A sutra-grahaka is further described as an expert in alignment, a rekajna, one who knows "the line".[1]
It is extremely interesting to note that land surveying is an ancient procedure followed by our ancestors.
Mathematical References in Vedas
The existence of numbers, their usage, the decimal system being in vogue, the concept of infinity are unambiguously presented in the following examples.[3]
Ascending numbers
In the Krshna Yajurveda, Taittriya Aranyaka, interesting passages containing a sequence of ascending numbers appear in the context of offering venerations to Agni (Deity of Fire) as follows
सकृत्ते अग्ने नमः । द्विस्ते नमः । त्रिस्ते नमः । चतुस्ते नमः । पञ्चकृत्वस्ते नमः । दशकृत्वस्ते नम । शतकृत्वस्ते नमः । आसहस्रकृत्वस्ते नमः । अपरिमितकृत्वस्ते नमः । नमस्ते अस्तु मा मा हिँ सीः ३ (Tait. Aran. 4.69)
Meaning: O Agni, salutations unto you once, salutations twice, salutations thrice, ...(four, five times). Salutations ten times, hundred times, thousand times. Salutations unto you unlimited times. My venerations to you, never ever hurt me.
Powers of 10
The Taittriya Samhita, presents a list of powers of 10 starting from hundred (10²) to a trillion (10 power 12).
शताय स्वाहा सहस्राय स्वाहा । अयुताय स्वाहा नियुताय स्वाहा प्रयुताय स्वाहा । अर्बुदाय स्वाहा न्यर्बुदाय स्वाहा समुद्राय स्वाहा मध्याय स्वाहा । अन्ताय स्वाहा परार्धाय स्वाहा । (Tait. Samh. 7.2.20)[4]
Meaning: Hail to hundred, ... hail to hundred thousand... hail to hundred million, hail to trillion.
Odd and Even numbers
A list of odd numbers and multiples of four occurs in the Taittriya Samhita (4.7.11)[5]
एका च मे तिस्रश् च मे पञ्च च मे सप्त च मे नव च म एकादश च मे त्रयोदश च मे पञ्चदश च मे सप्तदश च मे नवदश च म...
मे चतस्रश् च मे ऽष्टौ च मे द्वादश च मे षोडश च मे विꣳशतिश् च मे चतुर्विꣳशतिश् च मे ऽष्टाविꣳशतिश् च मे द्वात्रिꣳशच् च मे...
A mention of an interesting number 3339 is seen in the Rigveda, third mandala
त्रीणि शता त्री सहस्राण्यग्निं त्रिंशच्च देवा नव चासपर्यन् । औक्षन् घृतैरस्तृणन्बर्हिरस्मा आदिद्धोतारं न्यसादयन्त ॥९॥ (Rig. Veda. 3.9.9)[6]
Here the number 3339 (त्रीणि शता त्री सहस्राण्यग्निं त्रिंशच्च देवा नव) = 33+303+3003 and is also close to 18 years (approx. period of eclipse cycle).
Multiples of 10
In the Rigveda, second mandala we find multiples of ten listed.
आ विंशत्या त्रिंशता याह्यर्वाङा चत्वारिंशता हरिभिर्युजानः । आ पञ्चाशता सुरथेभिरिन्द्रा ऽऽषष्ट्या सप्तत्या सोमपेयम् ॥५॥ (Rig. Veda. 2.18.5)
आशीत्या नवत्या याह्यर्वाङा शतेन हरिभिरुह्यमानः । अयं हि ते... (Rig. Veda. 2.18.6)[7]
Meaning: O Indra, please come with twenty, thirty, forty, horses... with sixty, seventy... carried by hundred horses.
Notion of Infinite (∞)
The following widely recited mantra refers to the notion of infinite.
ॐ पूर्णमदः पूर्णमिदं पूर्णात् पूर्णमुदच्यते । पूर्णस्य पूर्णमादाय पूर्णमेवावशिष्यते ॥
Relationship between Shulba and Yajnas
Yajnas are of two main classes: Nitya (obligatory) and Kamya (optional or performed with a special intent). Nitya yajnas are indispensible and not performing them will incur papam (sin) to the yajamana. The Kamya yajnas are, however, optional and those who do not have any specific aims to achieve any desired objects need not perform them. Every yajna must be made in an altar of prescribed shape and size. These sutras give a compilation of principles in geometry that were used in designing the altars (called vedi or citi) where the yajnas were to be performed. The platforms of the altars were built with burnt bricks and mud mortar. It is stated that even a slight irregularity and variation in the form and size of the chiti (Citi) will nullify the object of the whole ritual and may even lead to an adverse effect, accuracy was the key. So ancient adhvaryus took utmost care in building a chiti of right shape and size.[1]
The chitis had rich symbolic significance and their designs were often intricate. For instance, the Syenacit has the shape of a falcon in flight (a symbolic representation of the aspiration of soaring upward); the Kurmachit is shaped as a tortoise, with extended head and legs, the rathacakracit as a chariot wheel with spokes, and so on.[8]
Subject-matter of Shulbasutras
Baudhayana shulbasutras are considered to be the most ancient of the Shulbasutra texts. It also presents a very systematic and detailed treatment of several topics that are skipped in later texts.
| Topics | English Equivalent | |
|---|---|---|
| 1 | रेखामानपरिभाषा | Units of linear measurement |
| 2 | चतुरश्रकरणोपायः | Construction of squares, rectangles, etc |
| 3 | करण्यानयनम् | Obtaining the surds/theorem of the square og the diagonal |
| 4 | क्षेत्राकारपरिणामः | Transformation of geometrical figures |
| 5 | नानाविधवेदिविहरणम् | Plan for different sacrificial grounds (darsa, pasubandha, sutramani, agnistoma etc) |
| 6 | अग्नीनां प्रमाणक्षेत्रमानम् | Areas of the sacrificial agnis/vedis |
| 7 | इष्टकसङ्ख्यापरिमाणादिकथनम् | Specifying the number of bricks used on the construction of vedis including their sizes and shapes |
| 8 | इष्टकोपधाने रीत्यादिनिर्णयः | Choosing clay, sand, etc in making bricks |
| 9 | इष्टकोपधानप्रकारः | Process of manufacturing the bricks |
| 10 | श्येनचिदाद्याकारनिरुपणम् | Describing the shapes of syenachiti |
A brief explanation of a few topics dealt in the shulbasutras is given below
Approximation of Surds
Besides presenting the details related to the construction of altars - that generally possess a bilateral symmetry - the Shulbasutra texts also present different interesting approximations for surds. The motivation for presenting estimates of surds could be traced to the attempts of vedic priests
- to solve the problem of 'squaring a circle' and vice versa
- to construct a square whose area is n times the area of a given square, and so on
Determination of cardinal directions
Determining the exact east-west line at a given location is a prerequisite for all constructions, be it a residence, a temple, a vedi or yajna kunda. Finding the cardinal directions using a shanku has been described thus:[3]
समे शंङ्कु निखाय शंङ्कुसम्मितया रज्ज्वा मण्डलं परिलिख्य यत्र लेखयोः शंङ्क्वग्रच्छाया निपतति तत्र शंङ्कू निहन्ति सा प्राची । (Katy. Shul. Sutr. 1.2)
Fixing a pin (or gnomon) on levelled ground and drawing a circle with a cord measured by the gnomon, he fixes pins at points on the line of the circumference where the shadow of the tip of the gnomon falls. That gives the east-west line (prachi). Now, to answer the question about the necessity of such an experiment, instead of simply looking at the sunrise or sunset to determine the cardinal directions, the commentator Mahidhara observes:
... तस्य उदयस्थानानां बहुत्वात् प्रतिदिनं भिन्नत्वात् अनियमेन प्राची ज्ञातुं न शक्या। तस्मात् शङ्कुस्थापनेन प्राचीसाधनमुक्तम्। दक्षिणायने चित्रापर्यन्तमर्कोऽभ्युदेति। मेषतुलासङ्क्रात्यहे प्राच्यां शुद्धायामुदेति। ततोऽर्कात् प्रचीज्ञानं दुर्घटम्।
Meaning: Since the rising points are many, varying from day to day, the (cardinal) east point cannot be known (from the sunrise point). Therefore it has been prescribed that the east be determined by fixing a Shanku (शङ्कु)... therefore simply looking at the sun and determining the east is difficult.
Having obtained the prachi, getting udichi (the north-south line) correctly is extremely important for the construction of various vedis having bilateral symmetry.[3]
References
- ↑ 1.0 1.1 1.2 1.3 1.4 Datta. Bibhutibhusan, (1932) The Science of the Sulba. A Study in Early Hindu Geometry. Calcutta: The University of Calcutta
- ↑ Bag, A. K., (1979) Mathematics in Ancient and Medieval India. Varanasi: Chaukhambha Orientalia. (Pages 103 - 174)
- ↑ 3.0 3.1 3.2 3.3 3.4 3.5 Prof. K. Ramasubramaniam's Lectures - Vedas and Sulbasutras, Parts 1 and 2
- ↑ Taittriya Samhita (Kanda 7 Prapathaka 2)
- ↑ Taittriya Samhita (Kanda 4 Anuvaka 7)
- ↑ Rigveda Shakala Samhita (Mandala 3 Sukta 3)
- ↑ Rigveda Shakala Samhita (Mandala 2 Sukta 18)
- ↑ A. K. Dutta and M. S. Sriram. Mathematics and Astronomy in India before 300 BCE.