Indian sine tables

From Dharmawiki
Revision as of 11:06, 16 April 2021 by BharataPuru (talk | contribs)
Jump to navigation Jump to search

Indian sine tables were constructed and improved upon by several ancient Indian mathematicians including the authors of Surya Siddhanta and Āryabhaṭa. Earliest sine table is found in Surya Siddhanta and another text is the astronomical treatise Āryabhaṭīya which was composed during the fifth century by the Indian mathematician and astronomer Āryabhaṭa (476–550 CE), for the computation of the half-chords of certain set of arcs of a circle. The table found in Surya Siddhanta is a table (in modern terms) of values of R.sinθ where R is the Indian standard radius of 3438 minutes. Āryabhaṭa's table is also not a set of values of the trigonometric sine function in a conventional sense; it is a table of the first differences of the values of trigonometric sines expressed in arcminutes, and because of this the table is also referred to as Āryabhaṭa's table of sine-differences.[1][2]

Some believe that the Āryabhaṭa's table was the first sine table ever constructed in the history of mathematics.[3] Āryabhaṭa's table remained as the standard sine table of ancient India. There were continuous attempts to improve the accuracy of this table. These endeavors culminated in the eventual discovery of the power series expansions of the sine and cosine functions by Madhava of Sangamagrama (c.1350 – c.1425), the founder of the Kerala school of astronomy and mathematics, and the tabulation of a sine table by Madhava with values accurate to seven or eight decimal places.

Ancient Indian concepts of Jya (chord) and Chaap or Dhanu (arc)

Jyā, koti-jyā and utkrama-jyā are three trigonometric functions introduced by Indian mathematicians and astronomers. The earliest known Indian treatise containing references to these functions is Surya Siddhanta.[4] These are functions of arcs of circles and not functions of angles. Jyā and kotijyā are closely related to the modern trigonometric functions of sine and cosine. In fact, the origins of the modern terms of "sine" and "cosine" have been traced back to the Sanskrit words jyā and kotijyā.[4]

Jya Figure.jpg

Definition

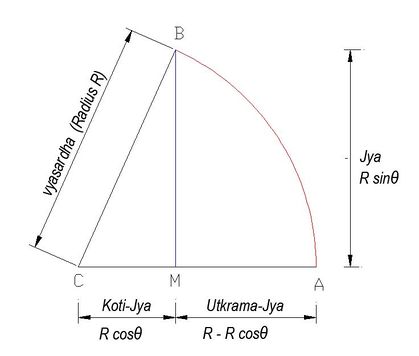
Let 'arc AB' denote an arc whose two extremities are A and B of a circle with center O. If a perpendicular BM be dropped from B to OA, then:

  • Vyāsardhā = Radius (R)
  • jyā of arc AB = MB
  • koti-jyā of arc AB = CM = R cos θ
  • utkrama-jyā of arc AB = MA

If the radius of the circle is R and the length of arc AB is s, the angle subtended by arc AB at O measured in radians is θ = s / R. The three Indian functions are related to modern trigonometric functions as follows:

  • jyā ( arc AB ) = R sin θ
  • koti-jyā ( arc AB ) = R cos θ
  • utkrama-jyā ( arc AB ) = R - R cos θ

An arc of a circle is like a bow and so is called a dhanu or cāpa which in Sanskrit means "a bow". The straight line joining the two extremities of an arc of a circle is like the string of a bow and this line is a chord of the circle. This chord is called a jyā which in Sanskrit means "a bow-string". The word jīvá is also used as a synonym for jyā in geometrical literature.[5] At some point, Indian astronomers and mathematicians realised that computations would be more convenient if one used the halves of the chords instead of the full chords and associated the half-chords with the halves of the arcs.[4][6] The half-chords were called ardha-jyās or jyā-ardhas. These terms were again shortened to jyā by omitting the qualifier ardha which meant "half of".

The Sanskrit word koṭi has the meaning of "point, cusp", and specifically "the curved end of a bow". In trigonometry, it came to denote "the complement of an arc to 90°". Thus koṭi-jyā is "the jyā of the complementary arc". In Indian treatises, especially in commentaries, koṭi-jyā is often abbreviated as kojyā. The term koṭi also denotes "the side of a right angled triangle". Thus koṭi-jyā is the base/Run of a right triangle with jyā being the perpendicular/rise .[4]

Utkrama means "inverted", thus utkrama-jyā means "inverted chord". The tabular values of utkrama-jyā are derived from the tabular values of jyā by subtracting the elements from the radius in the reversed order. This is really the arrow between the bow and the bow-string and hence it has also been called bāṇa, iṣu or śara all meaning "arrow".[4]

An arc of a circle which subtends an angle of 90° at the center is called a vritta-pāda (a quadrat of a circle). Each zodiacal sign defines an arc of 30° and three consecutive zodiacal signs defines a vritta-pāda. The jyā of a vritta-pāda is the radius of the circle. The Indian astronomers coined the term tri-jyā to denote the radius of the base circle, the term tri-jyā being indicative of "the jyā of three signs". The radius is also called vyāsārdha, viṣkambhārdha, vistarārdha, etc., all meaning "semi-diameter".[4]

According to one convention, the functions jyā and koti-jyā are respectively denoted by "Rsin" and "Rcos" treated as single words.[4] Others denote jyā and koti-jyā respectively by "Sin" and "Cos" (the first letters being capital letters in contradistinction to the first letters being small letters in ordinary sine and cosine functions).[6]

From jyā to sine

The origins of the modern term sine have been traced to the Sanskrit word jyā,[7][8] or more specifically to its synonym jīva. This term was adopted in medieval Islamic mathematics, transliterated in Arabic as jība (جيب). Since Arabic is written without short vowels – and as a borrowing the long vowel is here denoted with yāʾ – this was interpreted as the homographic jayb, which means "bosom". The text's 12th-century Latin translator used the Latin equivalent for "bosom", sinus.[9] When jyā became sinus, by analogy kojyā became co-sinus.

The Indian sine tables

Surya Siddhanta sine table

The Surya Siddhanta provides methods to calculate the sine value in chapter 2. It uses an Indian standard circle of radius 3438 units. It divides the quadrant into 24 equal segments with each segment sweeping an angle of 3.75° and a length of 225 minutes. The verse 15-16 translates as

The eighth part of the number of minutes contained in a zodiac sign (Rashi) (i.e. 1800) is the first sine (Jya). Divide the first sine by itself, subtract the quotient by that sine and add the remainder to that sine: the sum will be the second sine. In this manner divide successively the sines by the first sine, subtract the quotient from the divisor and add the remainder to the sine last found and the sum will be next sine. Thus you get twenty four sines (in a quadrant of a circle whose radius is 3438 units)[10]

The verse 17-22 translates as

The Twenty four sines are 225, 449, 671, 890, 1105, 1315, 1520, 1719, 1910, 2093, 2267, 2431, 2585, 2728, 2859, 2978, 3084, 3177, 3256, 3321, 3372, 3409, 3431, 3438.

Subtract the sines separately from 3438 in the inverse order, the remainders are the versed sines. [11]

The verse 23-27 translates as

The versed sines in a quadrant are 7, 29, 66, 117, 182, 261, 354, 460, 579, 710, 853, 1007, 1171, 1345, 1528, 1719, 1918, 2123, 2333, 2548, 2767, 2989, 3213, 3438.[12]

Āryabhaṭa's sine table

The stanza in Āryabhaṭiya describing the sine table is reproduced below: 

    मखि  भखि  फखि  धखि  णखि  ञखि  ङखि  हस्झ  स्ककि  किष्ग  श्घकि  किघ्व |
    घ्लकि  किग्र  हक्य  धकि  किच  स्ग  झश  ङ्व  क्ल  प्त  फ  छ  कला-अर्ध-ज्यास् ||

The second section of Āryabhaṭiya titled Ganitapādd a contains a stanza indicating a method for the computation of the sine table. There are several ambiguities in correctly interpreting the meaning of this verse. For example, the following is a translation of the verse given by Katz wherein the words in square brackets are insertions of the translator and not translations of texts in the verse.[13]

  • "When the second half-[chord] partitioned is less than the first half-chord, which is [approximately equal to] the [corresponding] arc, by a certain amount, the remaining [sine-differences] are less [than the previous ones] each by that amount of that divided by the first half-chord."

This may be referring to the fact that the second derivative of the sine function is equal to the negative of the sine function. Āryabhaṭa has chosen the number 3438 as the value of radius of the base circle for the computation of his sine table. The rationale of the choice of this parameter is the idea of measuring the circumference of a circle in angle measures. In astronomical computations distances are measured in degrees, minutes, seconds, etc. In this measure, the circumference of a circle is 360° = (60 × 360) minutes = 21600 minutes. The radius of the circle, the measure of whose circumference is 21600 minutes, is 21600 / 2π minutes. Computing this using the value π = 3.1416 known to Aryabhata one gets the radius of the circle as 3438 minutes approximately. Āryabhaṭa's sine table is based on this value for the radius of the base circle.

The values encoded in Āryabhaṭa's Sanskrit verse can be decoded using the numerical scheme explained in Āryabhaṭīya, and the decoded numbers are listed in the table below. In the table, the angle measures relevant to Āryabhaṭa's sine table are listed in the second column. The third column contains the list the numbers contained in the Sanskrit verse given above in Devanagari script. For the convenience of users unable to read Devanagari, these word-numerals are reproduced in the fourth column in ISO 15919 transliteration. The next column contains these numbers in the Hindu-Arabic numerals. Āryabhaṭa's numbers are the first differences in the values of sines. The corresponding value of sine (or more precisely, of jya) can be obtained by summing up the differences up to that difference. Thus the value of jya corresponding to 18° 45′ is the sum 225 + 224 + 222 + 219 + 215 = 1105. For assessing the accuracy of Āryabhaṭa's computations, the modern values of jyas are given in the last column of the table.

Comparing different sine tables

Surya Siddhanta is the earliest known text to contain the sine table. Aryabhata's sine table calculates the same sine values as calculated in the Surya Siddhanta. It is evident that several Indian mathematicians improved upon the sine tables. Aryabhata calculates the sine values which are same as the ones in Surya Siddhanta but Madhava has not rounded off the Jyā values and have given them further in minutes, seconds and thirds. Madhava's Jyā values derived into modern sine values provide astonishing accuracy upto 6 decimals places when compared with modern sine values.

Sl. No Angle
(in degrees,
arcminutes) 		Āryabhaṭa's
value of Jyā (R.sine) 		Surya Siddhanta value of Jyā (R.sine) Surya Siddhanta versed sines Modern value
of Jyā R.sine 		Madhava's sine values Madhava's derived sine values Modern sine values
   1 Template:Center Template:Center Template:Center Template:Center Template:Center
224ʹ 50ʹʹ 22ʹʹʹ
 0.06540314 0.06540313
   2 Template:Center Template:Center Template:Center Template:Center Template:Center
448ʹ 42ʹʹ 58ʹʹʹ
 0.13052623 0.13052619
   3 Template:Center Template:Center Template:Center Template:Center Template:Center
670ʹ 40ʹʹ 16ʹʹʹ
 0.19509032 0.19509032
   4 Template:Center Template:Center Template:Center Template:Center Template:Center
889ʹ 45ʹʹ 15ʹʹʹ
 0.25881900 0.25881905
   5 Template:Center Template:Center Template:Center Template:Center Template:Center
1105ʹ 01ʹʹ 39ʹʹʹ
 0.32143947 0.32143947
   6 Template:Center Template:Center Template:Center Template:Center Template:Center
1315ʹ 34ʹʹ 07ʹʹʹ
 0.38268340 0.38268343
   7 Template:Center Template:Center Template:Center Template:Center Template:Center
1520ʹ 28ʹʹ 35ʹʹʹ
 0.44228865 0.44228869
   8 Template:Center Template:Center Template:Center Template:Center Template:Center
1718ʹ 52ʹʹ 24ʹʹʹ
 0.49999998 0.50000000
   9 Template:Center Template:Center Template:Center Template:Center Template:Center
1718ʹ 52ʹʹ 24ʹʹʹ
 0.55557022 0.55557023
   10 Template:Center Template:Center Template:Center Template:Center Template:Center
2092ʹ 46ʹʹ 03ʹʹʹ
 0.60876139 0.60876143
   11 Template:Center Template:Center Template:Center Template:Center Template:Center
2266ʹ 39ʹʹ 50ʹʹʹ
 0.65934580 0.65934582
   12 Template:Center Template:Center Template:Center Template:Center Template:Center
2430ʹ 51ʹʹ 15ʹʹʹ
 0.70710681 0.70710678
   13 Template:Center Template:Center Template:Center Template:Center Template:Center
2584ʹ 38ʹʹ 06ʹʹʹ
 0.75183985 0.75183981
   14 Template:Center Template:Center Template:Center Template:Center Template:Center
2727ʹ 20ʹʹ 52ʹʹʹ
 0.79335331 0.79335334
   15 Template:Center Template:Center Template:Center Template:Center Template:Center
2858ʹ 22ʹʹ 55ʹʹʹ
 0.83146960 0.83146961
   16 Template:Center Template:Center Template:Center Template:Center Template:Center
2977ʹ 10ʹʹ 34ʹʹʹ
 0.86602543 0.86602540
   17 Template:Center Template:Center Template:Center Template:Center Template:Center
3083ʹ 13ʹʹ 17ʹʹʹ
 0.89687275 0.89687274
   18 Template:Center Template:Center Template:Center Template:Center Template:Center
3176ʹ 03ʹʹ 50ʹʹʹ
 0.92387954 0.92387953
   19 Template:Center Template:Center Template:Center Template:Center Template:Center
3255ʹ 18ʹʹ 22ʹʹʹ
 0.94693016 0.94693013
   20 Template:Center Template:Center Template:Center Template:Center Template:Center
3320ʹ 36ʹʹ 30ʹʹʹ
 0.96592581 0.96592583
   21 Template:Center Template:Center Template:Center Template:Center Template:Center
3371ʹ 41ʹʹ 29ʹʹʹ
 0.98078527 0.98078528
   22 Template:Center Template:Center Template:Center Template:Center Template:Center
3408ʹ 20ʹʹ 11ʹʹʹ
 0.99144487 0.99144486
   23 Template:Center Template:Center Template:Center Template:Center Template:Center
3430ʹ 23ʹʹ 11ʹʹʹ
 0.99785895 0.99785892
   24 Template:Center Template:Center Template:Center Template:Center Template:Center
3437ʹ 44ʹʹ 48ʹʹʹ
 0.99999997 1.00000000

See also

  1. ↑ Lua error in Module:Citation/CS1/Identifiers at line 47: attempt to index field 'wikibase' (a nil value).
  2. ↑ Lua error in Module:Citation/CS1/Identifiers at line 47: attempt to index field 'wikibase' (a nil value).
  3. ↑ J J O'Connor and E F Robertson (June 1996). "The trigonometric functions". Retrieved 4 March 2010.<templatestyles src="Module:Citation/CS1/styles.css"></templatestyles>
  4. ↑ 4.0 4.1 4.2 4.3 4.4 4.5 4.6 B.B. Datta and A.N. Singh (1983). "Hindu Trigonometry" (PDF). Indian Journal of History of Science. 18 (1): 39–108. Retrieved 1 March 2010.<templatestyles src="Module:Citation/CS1/styles.css"></templatestyles>
  5. ↑ According to lexicographers, it is a synonym also meaning "bow-string", but only its geometrical meaning is attested in literature. Monier-Williams, A Sanskrit Dictionary (1899): " jīvá n. (in geom. = jyā) the chord of an arc; the sine of an arc Suryasiddhanta 2.57"; jīvá as a generic adjective has the meaning of "living, alive" (cognate with English quick)
  6. ↑ 6.0 6.1 Glen Van Brummelen (2009). The mathematics of the heavens and the earth : the early history of trigonometry. Princeton University Press. pp. 95–97. ISBN 978-0-691-12973-0.<templatestyles src="Module:Citation/CS1/styles.css"></templatestyles>
  7. ↑ "How the Trig Functions Got their Names". Ask Dr. Math. Drexel University. Retrieved 2 March 2010.<templatestyles src="Module:Citation/CS1/styles.css"></templatestyles>
  8. ↑ J J O'Connor and E F Robertson (June 1996). "The trigonometric functions". Retrieved 2 March 2010.<templatestyles src="Module:Citation/CS1/styles.css"></templatestyles>
  9. ↑ Various sources credit the first use of sinus to either: See Merlet, A Note on the History of the Trigonometric Functions in Ceccarelli (ed.), International Symposium on History of Machines and Mechanisms, Springer, 2004
    See Maor (1998), chapter 3, for an earlier etymology crediting Gerard.
    See Katx, Victor (July 2008). A history of mathematics (in English) (3rd ed.). Boston: Pearson. p. 210 (sidebar). ISBN 978-0321387004.<templatestyles src="Module:Citation/CS1/styles.css"></templatestyles>
  10. ↑ Deva Shastri, Pundit Bapu (1861). Translation of the Surya Siddhanta. pp. 15–16.
  11. ↑ Deva Shastri, Pundit Bapu (1861). Translation of the Surya Siddhanta. pp. 16.
  12. ↑ Deva Shastri, Pundit Bapu (1861). Translation of the Surya Siddhanta. pp. 16.
  13. ↑ Cite error: Invalid <ref> tag; no text was provided for refs named Katz
Retrieved from ‘https://dharmawiki.org/index.php?title=Indian_sine_tables&oldid=129870’